The Limit

The value of a function as its input closer to some value is called the limit.

Introduction

The word “limit” has several meanings in English Language but one of its meanings is a value.

In calculus, the word “limit” is used to represent a value of the function as the input approaches some value. In a function, an input is a variable.

Let’s learn the concept of the limit from a simple understandable example.

Example

$2x+3$

It is a simple algebraic function and the variable $x$ represents the input of the function.

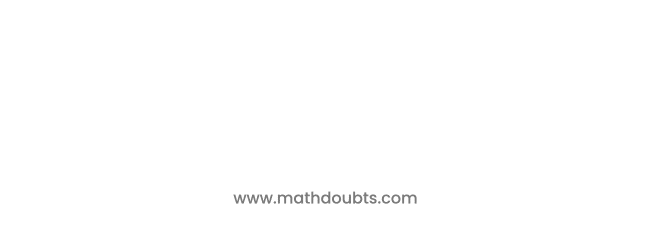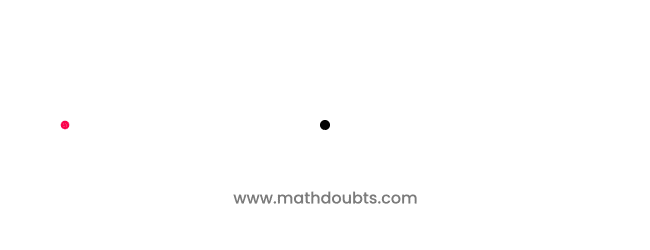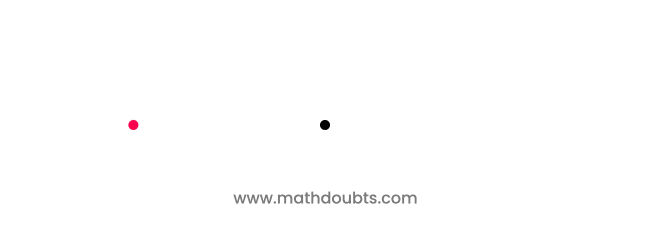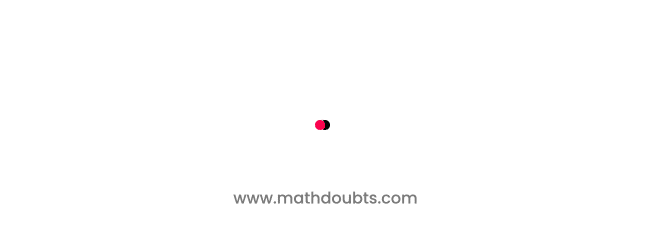
Let’s assume that we have to calculate the limit of the function as the variable $x$ closer to $5$.

We know that an operator for finding the limit of a function is simply denoted by $\lim$ and $x$ closer to $5$ is expressed as $x \,\to\, 5$ in calculus. Hence, the limit of the function $2x+3$ as $x$ approaches to $5$ is expressed in the following mathematical form.

$\displaystyle \large \lim_{x\,\to\,5}{\normalsize 2x+3}$

Remember that $x \,\to\, 5$ means we have to calculate the value of the function $2x+3$ by substituting a value, which is closer to $5$.

If x = 4

Firstly, find the difference between the numbers $4$ and $5$

$5-4 \,=\, 1$

There is no doubt about it that the number $4$ is near to $5$ but the difference between them is $1$, which indicates that there is some distance between them and it cannot be negligible. Hence, we cannot consider that the value of $4$ is closer to $5$.

If x = 4.9

Find the difference between the numbers $4.9$ and $5$.

$5-4.9 \,=\, 0.1$

$\implies$ $5-4.9 \,\approx\, 0$

In this case, the difference between $5$ and $4.9$ is $0.1$, which is approximately zero. Hence, we can take $4.9$ as a value closer to $5$. So, substitute $x \,=\, 4.9$ to find the limit of the function as $x$ approaches $5$.

$\displaystyle \large \lim_{x\,\to\,5}{\normalsize 2x+3}$ $\,=\,$ $2(4.9)+3$

$\implies$ $\displaystyle \large \lim_{x\,\to\,5}{\normalsize 2x+3}$ $\,=\,$ $9.8+3$

$\implies$ $\displaystyle \large \lim_{x\,\to\,5}{\normalsize 2x+3}$ $\,=\,$ $12.8$

$\,\,\,\therefore\,\,\,\,\,\,$ $\displaystyle \large \lim_{x\,\to\,5}{\normalsize 2x+3}$ $\,\approx\,$ $13$

Therefore, the limit of the function $2x+3$ as $x$ closer to $5$ is equal to $13$.

If x = 4.99

Once again, find the difference between $5$ and $4.99$.

$5-4.99 \,=\, 0.01$

$\implies$ $5-4.99 \,\approx\, 0$

The difference between $5$ and $4.99$ is $0.01$, which is also approximately zero. Therefore, we can take $4.99$ as a value closer to $5$. Hence, we can substitute $x = 4.99$ for evaluating the limit of the function as $x$ approaches $5$.

$\displaystyle \large \lim_{x\,\to\,5}{\normalsize 2x+3}$ $\,=\,$ $2(4.99)+3$

$\implies$ $\displaystyle \large \lim_{x\,\to\,5}{\normalsize 2x+3}$ $\,=\,$ $9.98+3$

$\implies$ $\displaystyle \large \lim_{x\,\to\,5}{\normalsize 2x+3}$ $\,=\,$ $12.98$

$\,\,\,\therefore\,\,\,\,\,\,$ $\displaystyle \large \lim_{x\,\to\,5}{\normalsize 2x+3}$ $\,\approx\,$ $13$

Therefore, the limit of the function $2x+3$ as $x$ tends to $5$ is equal to $13$.

Conclusion

Not only, $x = 4.9$ and $x = 4.99$. You can take any value which is closer to $5$ as input value to calculate the limit of the function $2x+3$. For example, $4.95$, $4.907$, $4.9999$ and so on.

Thus, we can calculate the limit of any function in the calculus.

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.