Slope of a Straight Line in terms of Coordinates of two points

Slope of a straight line can be expressed in terms of coordinates of any two points of the same straight line. It is also used to calculate the slope of the straight line but this method is actually used to calculate slope of the straight line when the angle, made by the straight line is unknown but the coordinates of any two points of the line is known.

Geometrical Explanation

Assume, AB↔ is a straight line in a plane and it makes an angle with horizontal axis in anticlockwise direction.

Now, draw a line from point A (parallel to horizontal axis) and also draw a line from point B (perpendicular to the horizontal axis). The lines drawn from point A and B intersect at a point, which is assumed to call point C. Thus, it forms a right angled triangle ΔBAC. The line segments AB‾, AC‾ and BC‾ become hypotenuse, adjacent side and opposite side of the right angled triangle ΔBAC. Assume, the angle between adjacent side and hypotenuse is θ. Observe, right angled triangle ΔBAC carefully. The angle of the right angled triangle is same as the angle made by the straight line AB↔.

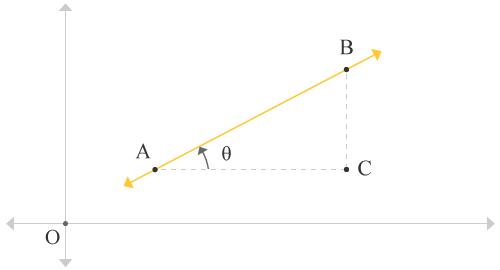
According to principle definition of slope of the straight line, the relation between slope of the straight line and angle made by the straight line is written in mathematics as follows.

The slope of the straight line is (m) = tanθ

Assume, the point A is located at (x1, y1) and also assume the point B is located at (x2, y2).

Observe the right angled triangle ΔBAC carefully.

The length of the opposite side is (BC) = OB – OC = y2  –  y1

The length of the adjacent side is (AC) = OC – OA = x2  –  x1

However, trigonometric function tangent can be expressed as ratio of opposite side to adjacent side. It is written in mathematical form as follows.

tanθ =BCAC = y2  –  y1x2  –  x1

We have known that slope of a straight line is m = tanθ

Now, replace the value of tangent to get the slope of straight line in terms of the coordinates of any two points of the straight line AB↔.

⇒ m = tanθ = y2  –  y1x2  –  x1

Therefore, the slope of the straight line AB↔ is (m) = y2  –  y1x2  –  x1

It is used as a mathematical formula to calculate the slope of any straight line when the angle made by the straight line is unknown but coordinates of any two points of the straight line is known.

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.