Expressing a straight line in algebraic form, contains the terms of any two points on same line is defined equation of the straight line in terms of any two points of the line.
In Geometric system, a straight line is usually represented by the any two points of the straight line and it can also be written in mathematical form in terms of same two points of the straight line. The equation of the straight line tells us what exactly the straight line is there on the plane of the coordinate geometric system.
Assume, there is straight line on a plane, and it is assumed to call straight line AB↔ and the straight line is having a slope by making an angle with horizontal x-axis.
The points A and B of the straight line are located at (x1, y1) and (x2, y2) geometrically in the Cartesian coordinate geometric system. Also assume, the angle made by the straight line AB↔ with horizontal axis is theta (θ).
Draw a straight line from A towards right hand side but it should exactly parallel to the horizontal x-axis. Similarly, draw a straight line from B downwards but it should exactly perpendicular to the same horizontal x-axis. Assume the line straight drawn from point A and the straight line drawn from point B are perpendicularly meet at a point, which is assumed to call point C. Thus, the straight line AB↔ is formed a right angled triangle ΔBAC. The angle made by the straight line AB↔ also acts as angle of the triangle.

In the right angled triangle ΔBAC, the line segments AB‾, BC‾ and AC‾ are known hypotenuse, opposite side and adjacent side of the right angled triangle.
Consider a point on the straight line AB↔ and assume to call it point D. Assume, the point D is located at (x, y) in the coordinate geometric system. Draw a perpendicular line to line segment AC↔ from point D and assume it intersects the line segment AC↔ at a point which is assumed to call point E. Thus, another right angle triangle, known ΔDAE is formed.
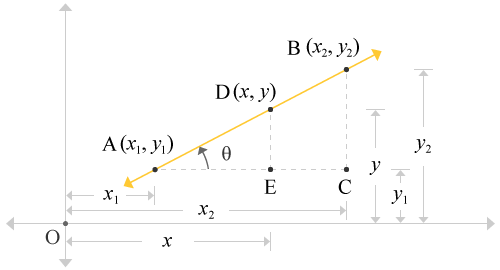
The triangle ΔDAE and ΔBAC are similar triangles because the angle made by the straight line is angle to the both right angled triangles.
According to the right angled triangle ΔDAE,
tanθ = DEAE
The length of the line segment (DE‾) is DE = OD – OE = y – y1
The length of the line segment (AE‾) is AE = OE – OA = x – x1
Therefore, tanθ = DEAE = (y – y1)(x – x1)
⇒ tanθ = (y – y1)(x – x1)
According to the right angled triangle ΔBAC,
tanθ = BCAC
The length of the line segment (BC‾) is BC = OB – OC = y2 – y1
The length of the line segment (AC‾) is AC = OC – OA = x2 – x1
Therefore, tanθ = BCAC = (y2 – y1)(x2 – x1)
⇒ tanθ = (y2 – y1)(x2 – x1)
The triangle ΔDAE is given a value for tanθ and the triangle ΔBAC has given another value for tanθ but they both are values for tanθ. So, they should be equal in value.
Therefore, tanθ = (y – y1)(x – x1) = (y2 – y1)(x2 – x1)
⇒ (y – y1)(x – x1) = (y2 – y1)(x2 – x1)
⇒ (y – y1)(x – x1) = (y2 – y1)(x2 – x1)
It is expressed in three different forms but all three represents equation of a straight line.
⇒ (y – y1)(x2 – x1) = (x – x1)(y2 – y1)
It is one form of the equation of the straight line in terms of the coordinates of the any two points of the line. It can also be written as follows. It is another form of this equation.
⇒ (y – y1) = (y2 – y1)(x2 – x1)(x – x1)
The ratio of difference of the y coordinates to difference of the x coordinates is known slope of the straight line. So, it can also be written as follows. It is the third form of the equation of the straight line.
⇒ (y – y1) = m(x – x1)
A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.
Copyright © 2012 - 2023 Math Doubts, All Rights Reserved