First Quadrant

The right-top side region in the two dimensional space is called the first quadrant.

Introduction

Two number lines are bisected perpendicularly at their centre point in two-dimensional Cartesian coordinate system for splitting the coordinate plane into four equal regions.

The right-top side region is called the first quadrant. The region in the angle $XOY$ is the first quadrant and represented by a Roman numeral $I$.

In $\angle XOY$, the $x$-axis and $y$-axis both represent positive values. Therefore, the signs of abscissa and ordinate of every point in this region must be positive.

If $x$-coordinate and $y$-coordinate of every point are represented by $x$ and $y$ respectively, then the values of them are written as $x > 0$ and $y > 0$ mathematically.

Usage

The first quadrant is used to identity the location of a point whose abscissa and ordinate are positive. Now, let’s learn how to use the first quadrant in the coordinate geometry.

Example

Identify the location of the point $A(4, 3)$.

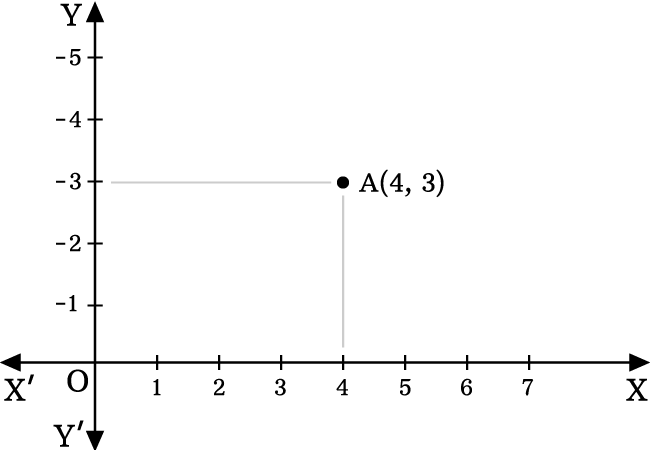
The $x$ coordinate (or abscissa) is $4$ and $y$ coordinate (or ordinate) is $3$ in this example.

1. Identity $4$ on positive $x$-axis. Draw a line from $4$ but it should be parallel to positive $y$ axis and perpendicular to positive $x$ axis.
2. Identify $3$ on negative $y$ axis. Draw a line from $3$ but it should be perpendicular to positive $y$ axis and parallel to positive $x$ axis.
3. The two lines get perpendicularly intersected at a point in the plane and it is the point $A(4, 3)$.

In this way, the first quadrant of two dimensional Cartesian coordinate system is used for identifying the location of any point whose both abscissa and ordinate are positive.

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.