Evaluate $\sin{(45^\circ+x)}$ $+$ $\sin{(45^\circ-x)}$

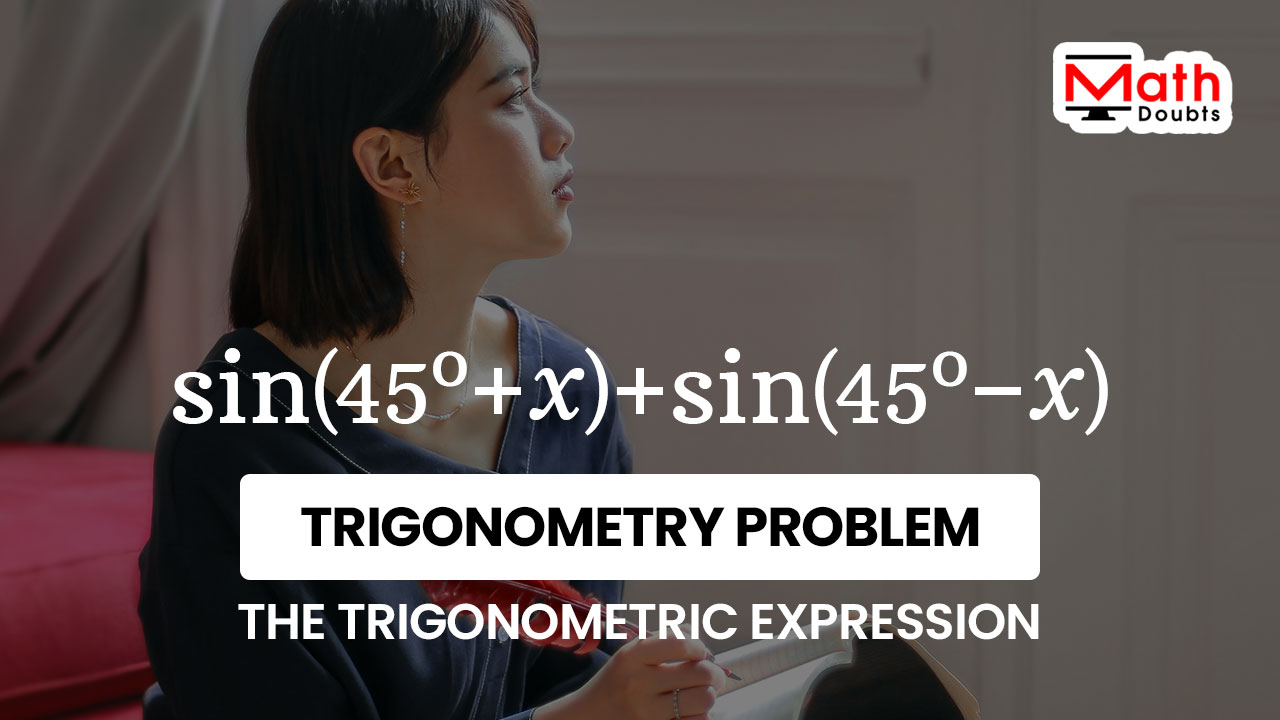
The sine of angle forty five degrees plus $x$ is added to sine of forty five degrees minus $x$, and sum of functions $\sin{(45^\circ+x)}$ and $\sin{(45^\circ-x)}$ should be evaluated in this trigonometry problem.

Expand the sine functions by identities

The sine of forty five degrees plus $x$ is a sum basis trigonometric function sine. So, it can be expanded by the angle sum identity of sine. Similarly, the sine of forty five degrees minus $x$ is a difference basis trigonometric function sine. So, it can be expanded by the angle difference identity of sine.

$=\,\,$ $\sin{(45^\circ)}\cos{x}$ $+$ $\cos{(45^\circ)}\sin{x}$ $+$ $\sin{(45^\circ)}\cos{x}$ $-$ $\cos{(45^\circ)}\sin{x}$

Simplify the Trigonometric expression

The two terms trigonometric expression is expanded as a four term trigonometric expression. Now, compare every term with remaining terms and we observe that the second and fourth trigonometric terms are same. So, the places of terms in the trigonometric expression can be changed by the commutative property of addition.

$=\,\,$ $\sin{(45^\circ)}\cos{x}$ $+$ $\sin{(45^\circ)}\cos{x}$ $+$ $\cos{(45^\circ)}\sin{x}$ $-$ $\cos{(45^\circ)}\sin{x}$

It is time to start the simplification process to evaluate the trigonometric expression. The first two terms are same and the remaining two terms are also same. There is a plus sign between the first two terms. So, the like terms can be added. There is a negative sign between the remaining two terms. So, they both get cancelled.

$=\,\,$ $2\sin{(45^\circ)}\cos{x}$ $+$ $\cancel{\cos{(45^\circ)}\sin{x}}$ $-$ $\cancel{\cos{(45^\circ)}\sin{x}}$

$=\,\,$ $2\sin{(45^\circ)}\cos{x}$

Find the value of the Trigonometric expression

The trigonometric binomial is simplified as a trigonometric monomial. Now, let’s evaluate the trigonometric expression to find the value of the given trigonometric expression.

$=\,\,$ $2 \times \sin{(45^\circ)} \times \cos{x}$

According to trigonometry, the sin 45 degrees value is equal to $1$ divided by square root of $2$.

$=\,\,$ $2 \times \dfrac{1}{\sqrt{2}} \times \cos{x}$

It is time to simplify the trigonometric expression further for evaluating the given trigonometric expression mathematically. Firstly, multiply the first two factors by the multiplication of the fractions.

$=\,\,$ $\dfrac{2 \times 1}{\sqrt{2}} \times \cos{x}$

$=\,\,$ $\dfrac{2}{\sqrt{2}} \times \cos{x}$

The square root of $2$ is there in the denominator. It is better to write the numerator in the form of denominator. The square of the $\sqrt{2}$ is equal to $2$. Hence, the number $2$ can be written in the form of the square root of $2$.

$=\,\,$ $\dfrac{\big(\sqrt{2}\big)^2}{\sqrt{2}} \times \cos{x}$

Now, let us focus on simplifying the above trigonometric expression.

$=\,\,$ $\dfrac{\cancel{\big(\sqrt{2}\big)^2}}{\cancel{\sqrt{2}}} \times \cos{x}$

$=\,\,$ $\sqrt{2} \times \cos{x}$

$=\,\,$ $\sqrt{2}\cos{x}$

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.