Evaluate $(\sec{x}+\tan{x})$ $\bigg(\dfrac{1-\sin{x}}{\cos{x}}\bigg)$

The product of the secant of angle $x$ plus tangent of angle $x$, and the quotient of one minus sine of angle $x$ divided by cosine of angle $x$ should be evaluated by simplifying the trigonometric expression in this trigonometry problem.

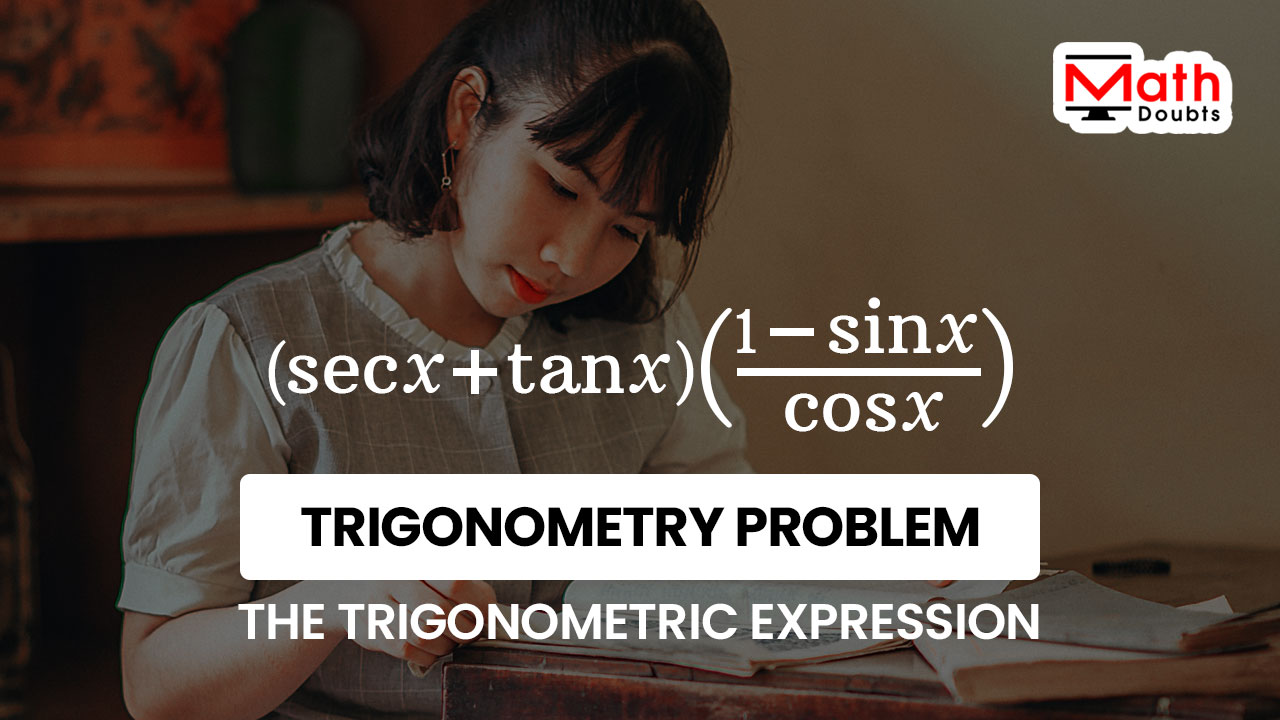
Let us learn how to evaluate the $\sec{x}$ plus $\tan{x}$ times $1$ minus $\sin{x}$ divided by $\cos{x}$ by simplifying this trigonometric expression.

Convert the functions into Sine and cosine

There are two factors in the trigonometric expression. One factor is expressed in terms of secant and tangent functions, but the second factor is expressed in terms of sine and cosines functions. So, it is better to express the terms in the first factor in terms of sine and cosine.

According to the reciprocal of cosine identity, the secant function $\sec{x}$ can be written as the reciprocal of cosine function $\cos{x}$. The tan function $\tan{x}$ can be expressed as the quotient of sine function $\sin{x}$ divided by cosine function $\cos{x}$ as per the quotient identity of sine and cosine.

$=\,\,$ $\bigg(\dfrac{1}{\cos{x}}+\dfrac{\sin{x}}{\cos{x}}\bigg)$ $\bigg(\dfrac{1-\sin{x}}{\cos{x}}\bigg)$

Find the sum of Fractions by the addition

There are two fractions in the first factor and they both are connected by a plus sign. So, let us add them to find their sum by the addition rule of the fractions.

$=\,\,$ $\bigg(\dfrac{1+\sin{x}}{\cos{x}}\bigg)$ $\bigg(\dfrac{1-\sin{x}}{\cos{x}}\bigg)$

Find the Product by Multiplying the fractions

Two fractions in terms of sine and cosine functions involved in multiplication and their product can be evaluated by multiplying the trigonometric fractions as per the multiplication rule of the fractions.

$=\,\,$ $\dfrac{(1+\sin{x})}{\cos{x}}$ $\times$ $\dfrac{(1-\sin{x})}{\cos{x}}$

$=\,\,$ $\dfrac{(1+\sin{x}) \times (1-\sin{x})}{\cos{x} \times \cos{x}}$

In the numerator, there are two binomials with common functions $1$ and $\sin{x}$ but they are connected with opposite mathematical signs and the product of them can be calculated as the difference of their squares as per the difference of squares identity.

In the denominator, the cosine function $\cos{x}$ is multiplied by itself. So, the product of two cosine of angle $x$ functions can be written in exponential notation as their product.

$=\,\,$ $\dfrac{1^2-\sin^2{x}}{\cos^2{x}}$

Evaluate Trigonometric expression by simplifying

The square of one is equal to one. Now, let us focus on finding the value of the trigonometric expression by simplifying the trigonometric expression in rational form.

$=\,\,$ $\dfrac{1-\sin^2{x}}{\cos^2{x}}$

According to the cos square identity, the square of cos function can be expanded as the subtraction of the square of sine square function from one. Therefore, one minus sine squared of angle $x$ can be simplified as cos squared of angle $x$.

$=\,\,$ $\dfrac{\cos^2{x}}{\cos^2{x}}$

$=\,\,$ $\dfrac{\cancel{\cos^2{x}}}{\cancel{\cos^2{x}}}$

$=\,\,$ $1$

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.